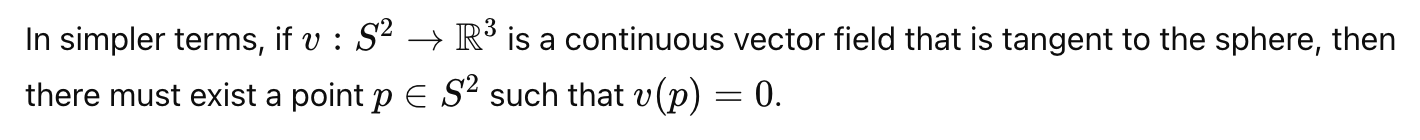The Hairy Ball Theorem
A Twisted Reality in Mathematics
Picture a ball completely covered in hair, and your challenge is to smooth all the hair so that it lies flat across the entire surface. If this ball were shaped like a bagel or existed in a two-dimensional world, the task might seem straightforward. However, in our three-dimensional reality, you'll quickly find yourself in a tangled mess—quite literally.
This scenario isn't just a quirky hypothetical; it illustrates a profound mathematical principle called the Hairy Ball Theorem. And yes, that’s its actual name. This theorem, grounded in algebraic topology, reveals something extraordinary: no matter how you attempt to comb the hair, there will always be at least one spot where a hair sticks up or points in a different direction.
The Mathematics Behind the Hairy Ball Theorem
Technically, the hairy ball theorem states that "a continuous vector field tangent to a sphere must have at least one point where the vector is zero." But what does that mean?
Let's unpack this. A vector field is simply a function that assigns a vector to every point in a space. For instance, imagine each hair on the ball as a vector that points in the direction the hair is lying. If you were to try to smooth out all these vectors so that they lie flat across the surface of the sphere, you'd find that it’s impossible to do so without encountering a point where the vector is zero—meaning the hair must stick straight up.
To visualize this, think of the Earth. The velocity of wind at any point on the Earth's surface can be represented as a vector field. The hairy ball theorem guarantees that, no matter what, there's always at least one point on Earth where the wind isn’t blowing—like the eye of a storm, where the wind speed is zero.
Examples in Real Life: The Wind on Earth
One of the most fascinating applications of the Hairy Ball Theorem is in meteorology. The Earth's surface can be modeled as a sphere, and the wind patterns form a vector field. According to the Hairy Ball Theorem, there must be at least one point on Earth where the wind speed is exactly zero. This point is where all the vectors (wind directions) cancel out.
This phenomenon is not just a theoretical curiosity; it's a fact of nature. The presence of cyclones, where air circulates around a point with calm at the center, is a manifestation of this theorem.
Equations and Formal Proof
To delve deeper, let’s consider the formal statement of the theorem:
There is no non-vanishing continuous tangent vector field on S2.
This theorem can be proved using the tools of algebraic topology, specifically through the use of the Poincaré-Hopf theorem, which relates the Euler characteristic of a surface to the indices of vector fields. Since the Euler characteristic of a sphere is 2, and every vector field must have indices summing to this characteristic, it implies the existence of at least one zero vector, i.e., a point where the hair sticks up or points in a different direction.
Concluding Thoughts: Beyond the Ball
What makes the hairy ball theorem so intriguing is that it extends beyond the playful imagery of a combed ball. It touches on fundamental aspects of topology, a branch of mathematics concerned with the properties of space that are preserved under continuous transformations. Whether you’re studying fluid dynamics, electrical fields, or even certain biological processes, the hairy ball theorem serves as a reminder of the elegant constraints imposed by the geometry of our universe.
So, the next time you find yourself pondering the patterns of the wind, or even combing your own hair, remember: sometimes, no matter how hard you try, there’s always that one stubborn strand that just won’t lie flat.
Unlock the Mysteries of Mathematics with Personalized Tutoring
Intrigued by the fascinating world of mathematical theorems and their real-world applications? Whether you're struggling to grasp complex concepts or looking to deepen your understanding, personalized tutoring can make all the difference. Together, we can explore the wonders of science and mathematics, from the basics to advanced topics like the Hairy Ball Theorem, and beyond.
Ready to take your math skills to the next level? Don’t let confusion hold you back. Contact me today to schedule a tutoring session tailored to your needs and start mastering science with confidence!
Thank you for reading!
-ScienceCourseGuy